Abstract
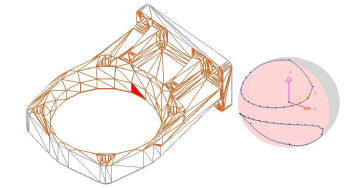
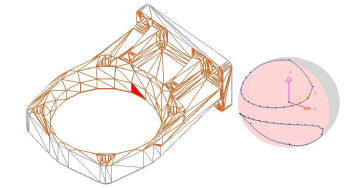
A global visibility map is a spherical image built to describe the complete set of global visible view directions for a surface. In this paper, we consider the computation of global visibility maps for regions on the boundary of a polyhedron. Both the self-occlusions introduced by a region and the global occlusions introduced by the rest of the surfaces on the boundary of the polyhedron are considered for computing a global visibility map. We show that the occluded view directions introduced between a pair of polyhedral surfaces can be computed from the spherical projection of the Minkowski sum of one surface and the reflection of the other. A suitable subset of the Minkowski sum, which shares the identical spherical projection with the complete Minkowski sum, is constructed to obtain the spherical images representing global occlusions. Our method has been successfully tested on many CAD models. It extends the previous methods for computing global visibility maps using convex decomposition, and it exhibits a better performance.